CONIC SECTIONS
Definition
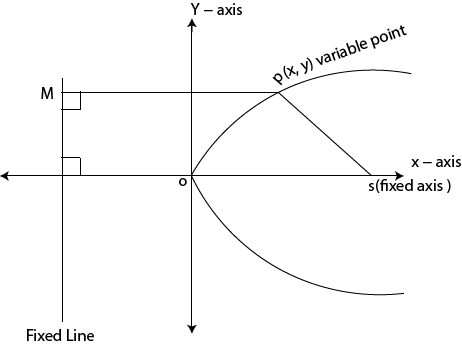
Conic sections or conics are the sections whose ratios of the distance of the variables point from the fixed point to the distance, or the variable point from the fixed line is constant
TYPES OF CONIC SECTION
There are i) Parabola
ii) Ellipse
iii) Hyperbola
IMPORTANT TERMS USED IN CONIC SECTION
I. FOCUS
This is the fixed point of the conic section.
For parabola
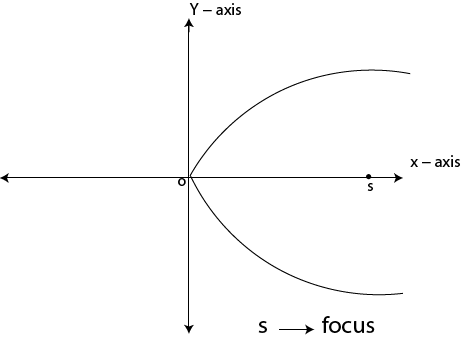
S → focus
For ellipse
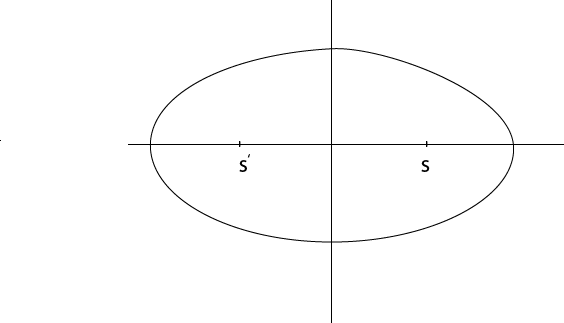
S and S’ are the foci of an ellipse
II. DIRECTRIX
This is the straight line whose distance from the focus is fixed.
For parabola
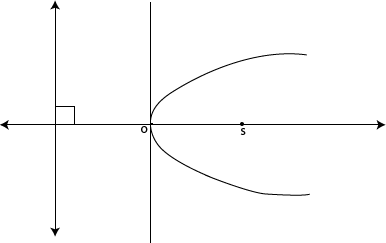
For ellipse
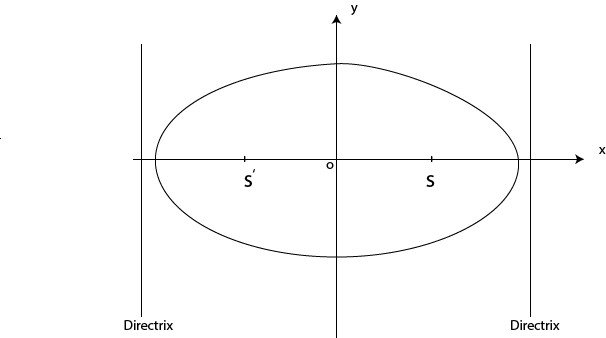
III. ECCENTRICITY (e)
This is the amount ratio of the distance of the variable point from the focus to the distance of the variables point from the directrix.
For Parabola
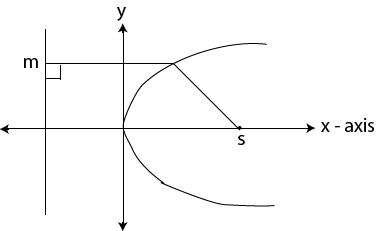
For ellipse
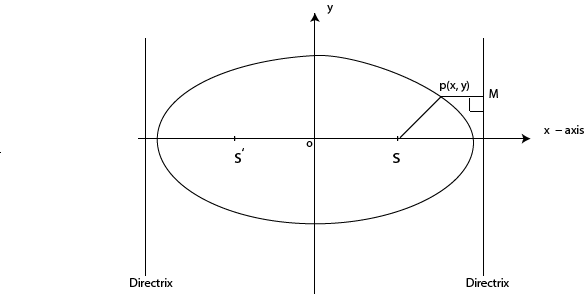
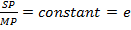
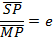
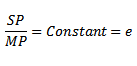
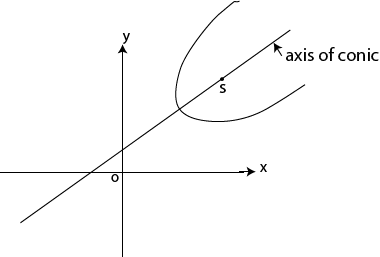
IV. AXIS OF THE CONIC
This is the straight line which cuts the conic or conic section symmetrically into two equal parts.
For parabola
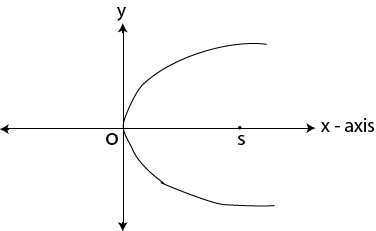
X-axis is the point of the conic i.e. parabola
Also
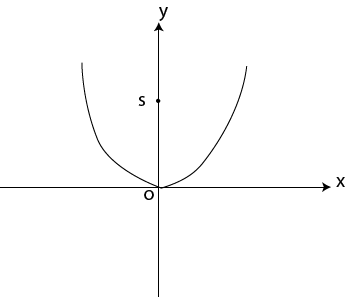
Y-axis is the axis of the conic
FOR ELLIPSE
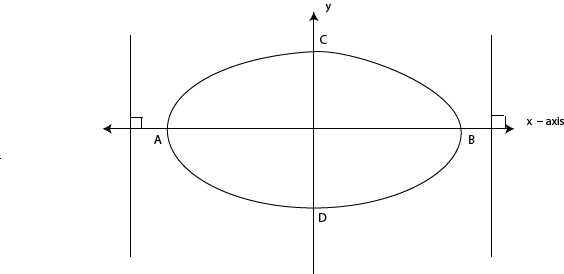
AB – is the axis (major axis) of the Conic i.e. (ellipse)
CD – is the axis (minor axis) the Conic i.e. (ellipse)
→An ellipse has Two axes is major and minor axes
V FOCAL CHORD
This is the chord passing through the focus of the conic section.
For parabola

For ellipse
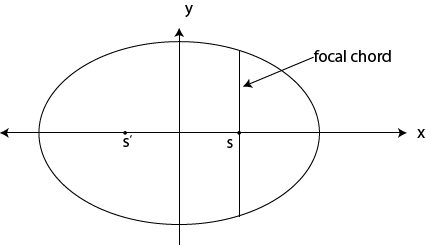
VI LATUS RECTRUM
This is the focal cord which is perpendicular to the axis of the conic section.
For parabola
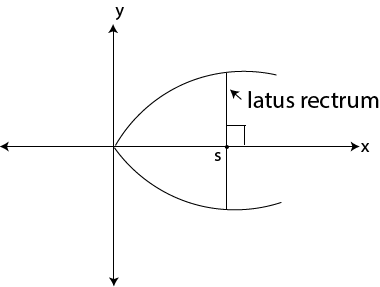
For Ellipse
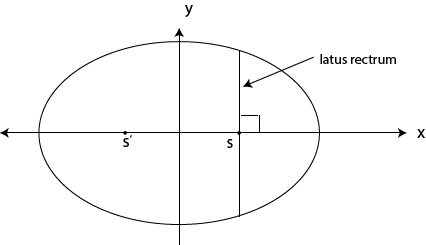
Note:
Latus rectum is always parallel to the directrix
VII. VERTEX
This is the turning point of the conic section.
For parabola
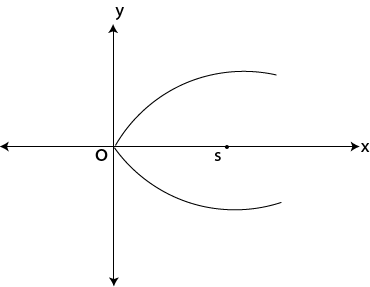
0 – is the vertex
For ellipse
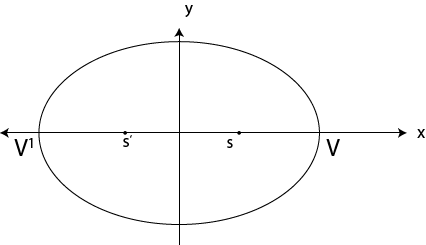
Where V and V1 is the vertex of an ellipse
PARABOLA
This is the conic section whose eccentricity, e is one i.e. e = 1

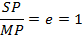
For parabola
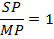
SP = MP
EQUATIONS OF THE PARABOLA
These are;
a) Standard equation
b) General equations
A. STANDARD EQUATION OF THE PARABOLA
1st case: Along the x – axis
· Consider the parabola whose focus is S(a, 0) and directrix x = -a
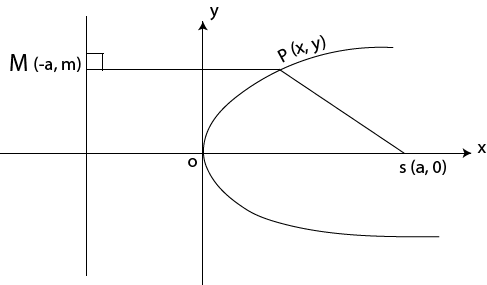
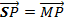
Squaring both sides
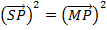
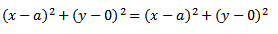
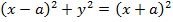
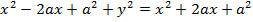
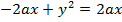
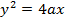
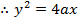
Is the standard equation of the parabola
PROPERTIES
i) The parabola lies along x – axis
ii) Focus, s (a, o)
iii) Directrix x = -a
iv) Vertex (0, 0) origin
Note:
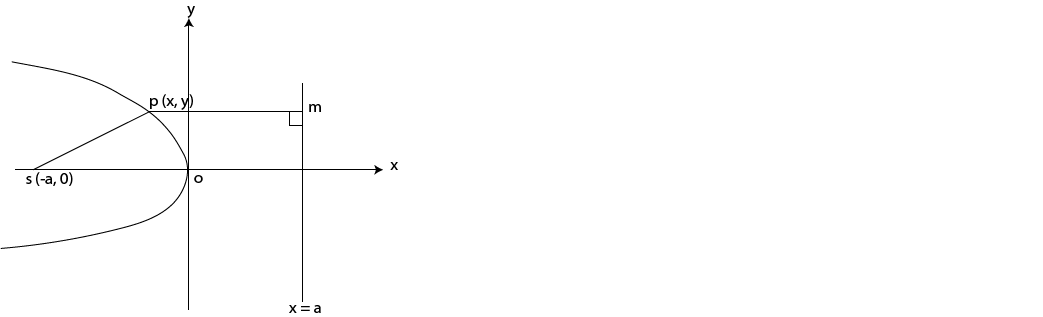
PROPERTIES
1) The parabola lies along x – axis
2) Focus s (-a, o)
3) Directrix x = a
4) Vertex (o, o) origin
2nd case: along y – axis
Consider the parabola when focus is s (o, a) and directrix y = -a
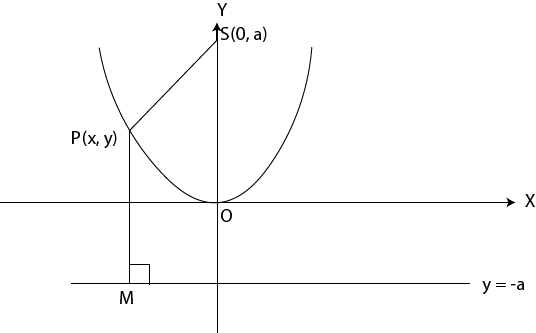
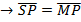
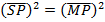
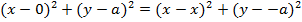
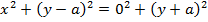
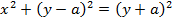
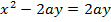
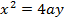
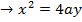
· Is the standard equation of the parabola along y – axis
PROPERTIES
i) The parabola lies along y – axis
ii) The focus s (o, a)
iii) Directrix y = -a
iv) Vertex (o, o) origin
Note;
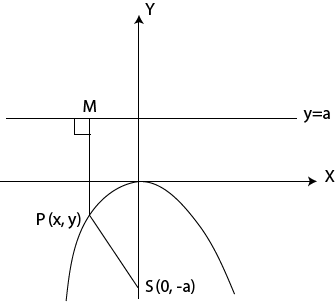
Hence, x2 = -4ay
PROPERTIES
i) The parabola lies along y – axis
ii) Focus s (o, -a)
iii) Directrix y = a
iv) Vertex (o, o)