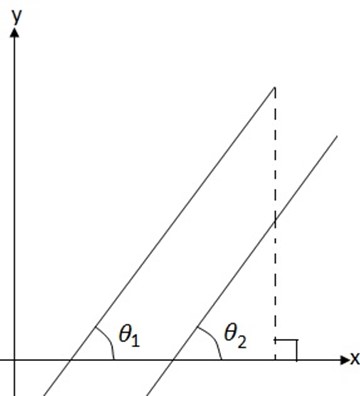
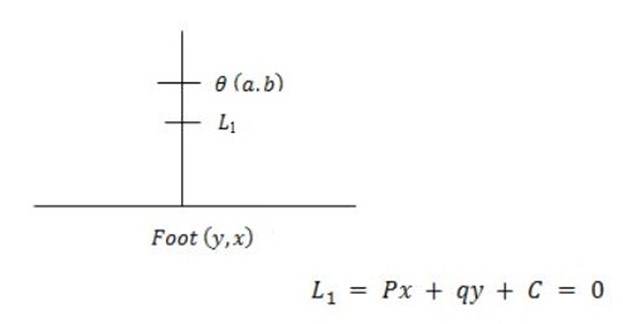ANGLE BETWEEN TWO LINES
-Let consider 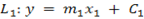 and
and 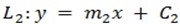 where Q is angle made between
where Q is angle made between 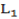 and
and 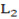 and
and 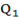 is the angle of
is the angle of 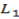 and
and 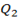 is the angle of
is the angle of  .
.
-Consider the figure below
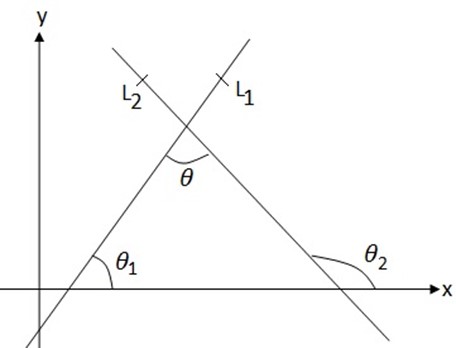
Our intentions to find the value of 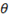 which always should be acute angle.
which always should be acute angle.
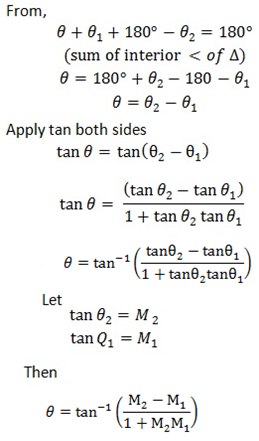
where
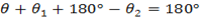
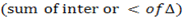
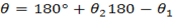
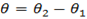
PARALLEL AND PERPENDICULAR LINES
(a) PARALLEL LINES
-Are the lines which never meet when they are produced
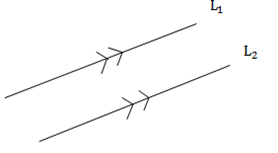
Means that  is parallel to
is parallel to  symbolically
symbolically  //
//
– -However condition for two or more lines to be parallel state that they posses the same gradient.
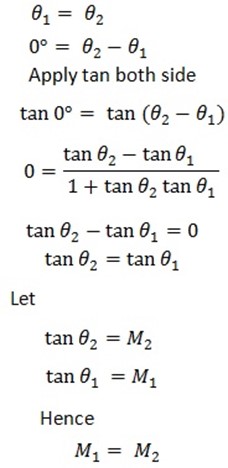
b) PERPENDICULAR LINES
– -Are the lines which intersect at right angle when they are produced.
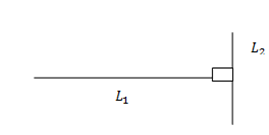
Means that 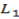 is perpendicular to
is perpendicular to 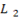
– -Symbolically is denoted as L1⊥L2
– -However the condition for two or more lines to be perpendicular states that “The product of their slopes should be equal to negative one”.
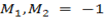
– -Let consider the figure below
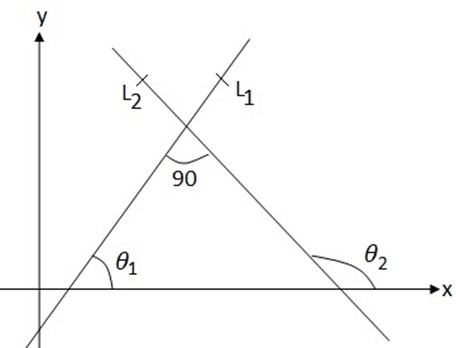
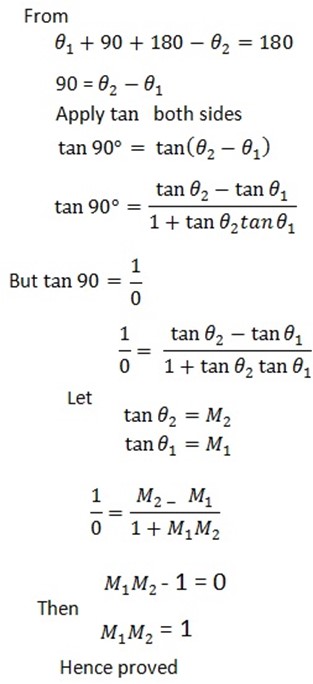
NOTE:
1. The equation of the line parallel to the line 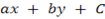 = 0 passing through a certain point is of the form of
= 0 passing through a certain point is of the form of 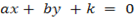 . Where
. Where 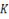 – is constant.
– is constant.
2. The equation of the line perpendicular to the line 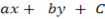 pass through a certain point is of the form of
pass through a certain point is of the form of 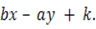 when
when 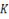 – is constant.
– is constant.
3. The calculation of K above done by substitution certain point passing through.
THE EQUATION OF PERPENDICULAR BI SECTOR
– Let two point be A and B.
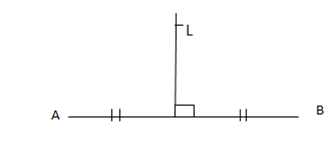
Where,
Line L is perpendicular bisector between point A and B.
Now our intention is to find the equation of L.
IMPORTANT STEPS
1. Determine the midpoint between point A and B.
2. Since L and  are ⊥ to each other then find slope of L.
are ⊥ to each other then find slope of L.
for
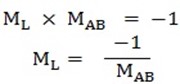
3. Get equation of L as equation of perpendicular bisector of 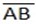 by using
by using 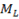 and mid point of A and B.
and mid point of A and B.
THE COORDINATE OF THE FOOT OF PERPENDICULAR FROM THE POINT
THE POINT TO THE LINE
– -Our intention is to find the coordinate of the foot (x,y) which act as the point if intersection of 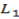 and
and 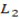 .
.
– Let consider the figure below.