Second order Differential Equations
Second order differential equation is of the form of;
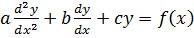
Where a, b, c are constant coefficients and 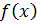 is a given function of x
is a given function of x
If 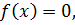 the equation is homogeneous otherwise it is a non-homogeneous
the equation is homogeneous otherwise it is a non-homogeneous
Which of the following are linear H.D.Es
1. 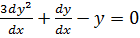
2. 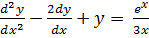
3. 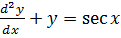
4. 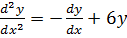
5. 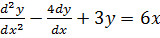
Characteristic (Auxiliary) Equation for H.DE
Consider a linear non-homogeneous 2nd order D.E
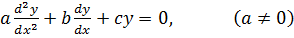
Let y = u and y=v be two solution of the equation
Where u and v a functions of x

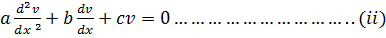
Adding (i) & (ii) gives
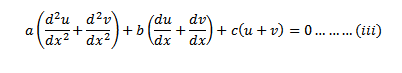
Then,
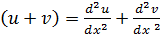 and
and
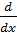
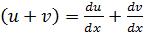
Becomes
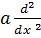
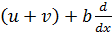
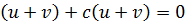
If y = u and y = v are the solutions of the equation
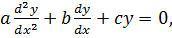
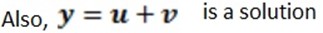
Suppose a = 0
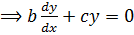
i.e. 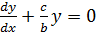
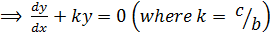
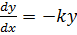 (separable)
(separable)
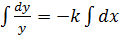
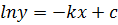
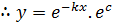
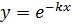 (where
(where 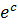 is constant)
is constant)
Take m for –k
– 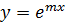 is the solution of dy +ky
is the solution of dy +ky
Also will be the solution of the equation
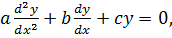 if it satisfies the equation
if it satisfies the equation
If 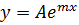
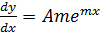
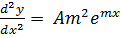
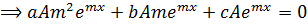
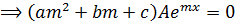
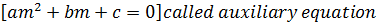
The values will be 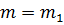 and
and 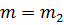
If 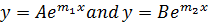 are two solution
are two solution
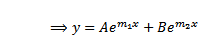
Note that:
If the Auxiliary equation has
(i) Two real roots 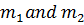
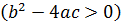
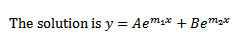
(ii) Equal roots 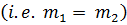
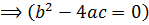
The solution is 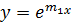
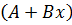
(iii) 2 Complex roots to the auxiliary equation
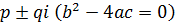
The solution is 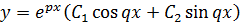
Examples
Solve the following 2nd order Des
1. 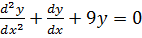
Solution
Auxiliary equation 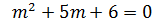
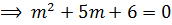
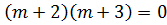
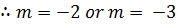
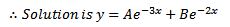
2. 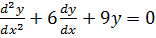
Solution
The auxiliary equation is
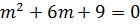
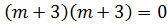
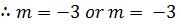
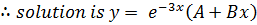
3. 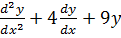
Solution
Auxiliary equation is
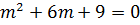
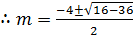
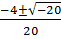
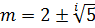
In this case P=-2 and q = 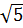
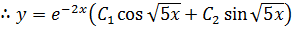
Exercise
Solve the following
1. 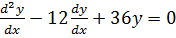
2. 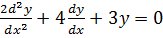
3. 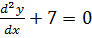
Non homogeneous 2nd order D.E
Consider the equation
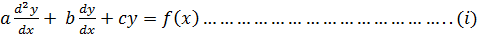
If 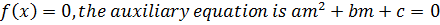
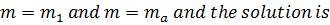
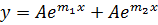
The general solution of (i) is given by
Note that
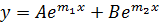 is called complementary function matas R.H.S Zero
is called complementary function matas R.H.S Zero
Y = f (x) is called particular intergral makes R.H.S 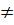 0
0
Consider the R.H.S function
i.e if 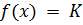 assume
assume 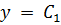
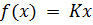
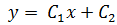




Examples
1. Solve 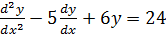
Solution
C.F solve L.H.S = 0
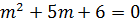
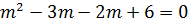
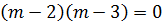
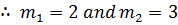
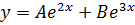
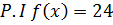
Assume 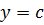
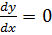
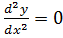
Substituting in the given equation gives
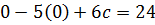
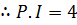
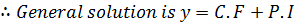
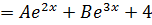
2. Solve 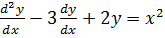
Solution
(i) C.F: 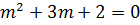
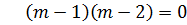
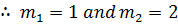
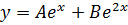
(ii) 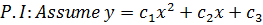
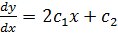
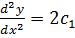
Substituting into the given equation
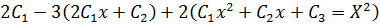
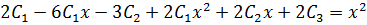
Collecting like terms
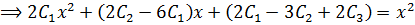
Comparing L.H.S to R.H.s
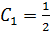
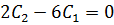
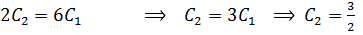
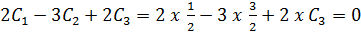
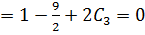

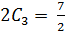
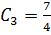
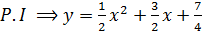
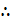 General solution
General solution
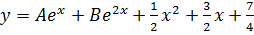
3. Solve 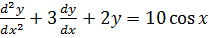
Given that 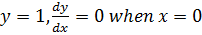
Solution
C.F: 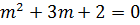
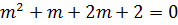
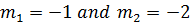
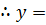
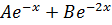
 I: Assume
I: Assume 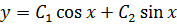
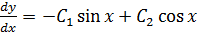
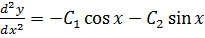
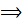
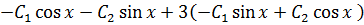 +2
+2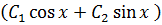
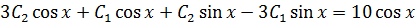
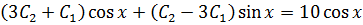
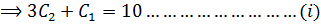
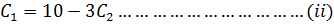
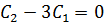
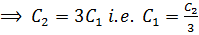
Substitute into (i)
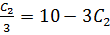
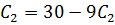
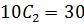
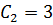
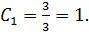
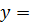
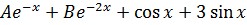
But y = 1, 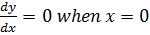
1= A + B + 1
A + B = 0
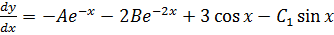
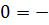 A-2B+3
A-2B+3
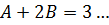 ………………………….(i)
………………………….(i)
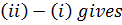
B = 3
Substituting into (ii) gives
A + 3 = 0
A = -3
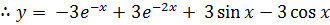
4. Solve 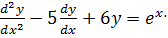
Solution
C.F: 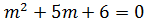
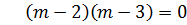
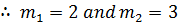
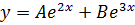

Let y = 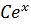
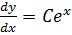
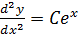
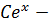 5
5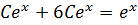
2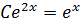
2C=1
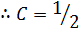
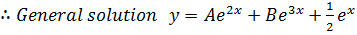
Note that: if P.I is contained in the C.F multiply the assumed P.I by x and go on
Example
Solve 
Solution
C.F: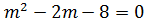
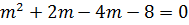
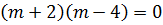
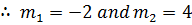
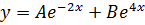
P.I: Assume y =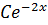
Since 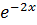 is already contained
is already contained 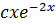
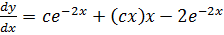
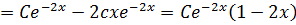
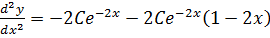
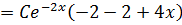
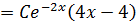
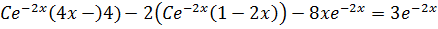
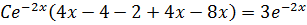
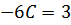
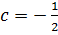
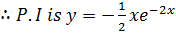
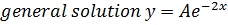 +B
+B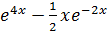
Exercise
Solve the following
(i) 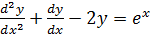
2nd order equations which are reducible to 1st order
Consider 2nd order equation which can not be written in the form
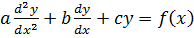
i.e 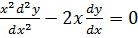
Such equation will be solved by the substitution of: –
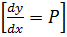
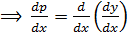
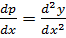
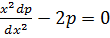
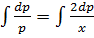
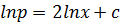
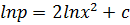
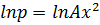
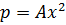
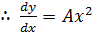
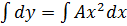
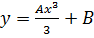
Where A and B are constants
Example
Solve 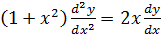
Solution
Let 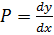
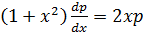
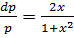 dx
dx
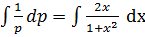
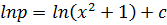
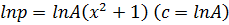
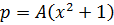
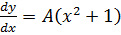
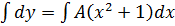
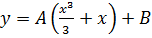
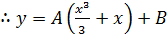
Example
Solve 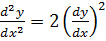
Solution
Let p = 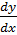
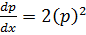
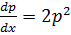
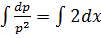
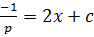
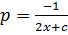
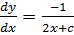
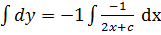
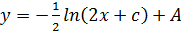
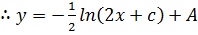
Example
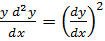
Solution
Let 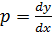
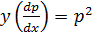
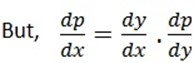
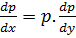
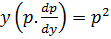
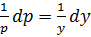
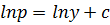
In p= ln Ay where c= ln A
P = Ay
i.e. 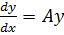

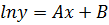
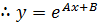
Note that:
– If P.I is contained in C.F, and C.F is of real roots Assume 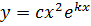 if
if 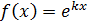
– If C.F is a distinct root, but one root is the same as that on P.I, assume 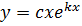 if
if 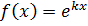
– If  , assume
, assume 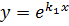 independently followed by
independently followed by 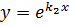 then add to obtained a P.I
then add to obtained a P.I