BAM FORM 5 – DIFFERENTIATION

HOW TO DETERMINE THE TYPE OF CRITICAL POINT

By testing the sign of the gradient on either sides of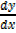  

By finding the second derivative of the function

If d²y / dx² is [+ve] we have the minimum point

If d²y / dx² is [-ve] we have maximum point

If d²y  =  0 we have either maximum, minimum or point or inflexion
dx²

Examples

i)    y = x² + 4x + 3

i.)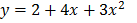    

iii.)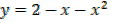   

Solution

y = x² + 4x +3

dy/ dx = 2x +4

When dy / dx = 0

2x +4 = 0

2x = -4

x = -2

Testing the sign of gradient

The minimum value of y = (-2)² + 4(-2) + 3

y = 4 -8 + 3

                                          y   = -1

Alternatively

d²y / dx² = 2

Since

d²y / dx² are (+ve), the function has minimum value

When dy / dx = 0

y = (-2)² +4 (-2) + 3

y = 4-8+3

y = 4-5

y = -1

Solution

y =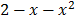   

dy / dx =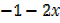  

When dy / dx = 0

 

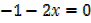
-2x = 1

y = – ½

Now d²y / dx² = -2[max pt]

The minimum value is

y = 2 – [- ½] – [-1/2] ²

y = 2+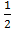– =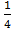