SEQUENCE
Is a set of numbers written in a definite order such that there is a rule by which the terms are obtained.
Or
Is a set of number with a simple pattern.
Example
1. A set of even numbers
• 2, 4, 6, 8, 10 ……
2. A set of odd numbers
• 1, 3, 5, 7, 9, 11….
Knowing the pattern the next number from the previous can be obtained.
Example
1. Find the next term from the sequence
• 2, 7, 12, 17, 22, 27, 32
The next term is 37.
2. Given the sequence
• 2, 4, 6, 8, 10, 12………
What is
i)The first term =2
ii) The 3rd term =6
iii)The 5th term =10
iv)The nth term [ the general formula]
2=2×1
4=2×2
6=2×3
8=2×4
10=2×5
12=2×6
Nth =2xn
Therefore nth term =2n
Find the 100th term, general formula =2n
100th term means n=100
100th term =2×100
100th term =200
3. Find the nth term
 ,
,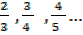
Solution
 ,
,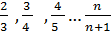
4. Given the general term 3[2n]
a) Find the first 5terms
b) Find the sum of the first 3 terms
Solution
3[2n] when;
n=1, 3 [21] =6
n=2, 3 [22] =12
n=3, 3 [23] =24
n=4, 3 [24] =48
n=5, 3 [25] =96
First 5 terms = 6, 12, 24, 45, 96
Sum of the three terms
Sum of the first three = 6+12+24
Sum of the first three = 42
Exercise 1.
1. Find the nth term of the sequence 1, 3, 5, 7…..
2. Find the nth term of the sequence 3, 6, 9, 12……
3. The nth term of a sequence is given by 2n+1 write down the 10th term.
4. The nth term of a certain sequence is 2n-1 find the sum of the first three terms.
5. If the general term of a certain sequence is 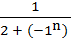
Find the first four terms increasing or
decreasing in magnitudes
Solution
1. 1, 3, 5, 7…nth
From the sequence the difference between the consecutive terms is 2 thus
nth =2+n
2. 3, 6, 9, 12……..nth
The difference between the consecutive terms is 3 thus
nth =3n
3. 10th = 2[10+1]
10th =20+1
10th =21
4. When
n=1, 21-1
n=2, 22-1
n=3, 23-1
Sum of the first three terms =1, 2, 4
Sum = 1+2+4
Sum = 7
5. When;
n = 1, 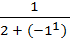 = 1
= 1
n = 2, 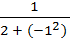 =
=
n = 3, 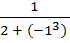 = 1
= 1
n = 4, 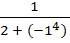 =
= 
The first four terms are 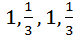
SERIES
Defination: When the terms of a sequence are considered as the sum, the expression obtained is called a serier or a propagation
Example
(a). 1+2+3+4+5+……….
(b). 2+4+6+8+10+………+100.
(c). -3-6-9-…….
The above expression represent a series. There are two types of series
1.Finite series
Finite series is the series which ends after a finite number of terms
e.g. 2+4+6+8+………….+100.
-3-6-9-12-………….-27.
2. infinite Series
Is a series which does not have an end.
e.g. 1+2+3+4+5+6+7+8…………..
1-1+1-1+1-1+1-1+1…………..
Exercise 5.2.1
1. Find the series of a certain sequence having 2(-1)n as the general term
2. Find the sum of the first ten terms of the series -4-1+2+…….
3. The first term of a certain series is k. The second term is 2k and the third is 3k. Find
(a). The nth term
(b). The sum of the first five terms
Exercise 5.2.1 Solution
1. n=1, 2(-1)1 = -2
n=2, 2(-1)2 = 2
n=3, 2(-1)3 = -2
n=4, 2(-1)4 = 2
n=5, 2(-1)5 = -2
The series is -2+2-2+2-2+2-2+2-2+2+………………..+2(-1)n
2. The sum of the first n terms of the series -4-1+2+5+8+11+14+17+20+23
= 95
3. (a) k + 2k + 3k + 4k +………. + nk
The nth term of the series is nk
(b) k + 2k + 3k + 4k + 5k = 15k
... The sum of the first 5 terms = 15k
ARITHMETIC PROGRESSION [A.P]
An arithmetic progression is a series in which each term differ from the preceding by a constant quantity known as the common difference which is denoted by “d“.
For instance 3, 6, 9, 12….. is an arithmetic progression with common difference 3.
The nth term of an arithmetic progression
If n is the number of terms of an arithmetic progression, then the nth term is denoted by An
Therefore An+1= An + d
e.g. First term = A1
Second term = A1+ d = A2
Third term = A2 + d = A3
Consider a series 3+6+9+12+…..
A1 = 3, d= 3
A2 = A1 + d
A3 = A2 + d = A1+d+d = A1+ 2d
A4 = A3 + d = A1+ 2d+ d = A1 + 3d
A5 = A4 + d = A1+ 3d+ d = A1 + 4d
A6 = A5 + d = A1+ 4d+ d = A1 + 5d
An = A[n-1] + d = A1+ (n-1)d
... The general formula for obtaining the nth term of the series is
An = A1+ (n-1)d
The general formula for obtaining the nth term in the sequence is also given by An =A1+[n-1]d
Question
1. A7 =  A2 and A4 =16. Find A1 and d.
A2 and A4 =16. Find A1 and d.
Solution
A7= A1+6d
=A1+6d= 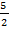 ( A1+d)
( A1+d)
2A1+12d=5A1+5d
3A1=7d
A1=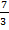 d
d
A 4 =16
A4=A1+3d = 16
 d +3d= 16
d +3d= 16
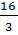 d = 16
d = 16
d= 3
But A1 +3d= 16
A1 +9=16
A1= 7
Exercise 2
1. The pth term of an A.P is x and the qth term of this is y, find the rth term of the same A.P
2. The fifth term of an A.P is 17 and the third term is 11. Find the 13th term of this A.P.
3. The second term of an A.P is 2 and the 16th term is -4 find the first term.
4. The sixth term of an A.P is 14 and the 9th of the same A.P is 20 find 10th term.
5. The second term of an A.P is 3 times the 6th term. If the common difference is -4 find the 1st term and the nth term
6. The third term of an A.P is 0 and the common difference is -2 find;
(a) The first term
(b) The general term
7. Find the 54th term of an A.P 100, 97, 94
8. If 4, x, y and 20 are in A.P find x and y
9. Find the 40th term of an A.P 4, 7, 10……..
10.What is the nth term of an A.P 4, 9, 14
11.The 5th term of an A.P is 40 and the seventh term of the same A.P. is 20 find the
a) The common difference
b) The nth term
12. The 2nd term of an A.P is 7 and the 7th term is 10 find the first term and the common difference
Exercise 2 Solution
1. d = y-x
rth = A1+[n-1]d
= A1+nd-d
= A1+n[y-x]-[y-x]
= A1+[ny-nx-[y-x]
=A1+[ny-y]-[nx-x]
A1+g[n-1]-x[n-1]
rth=A1+[y-x][n-1]
2.
A5=17
A3=11
A13=?
A5 =A1+4d = 17
A3=A1+2d =17
A1+2d=11
Solve the simultaneous equation by using equilibrium method.
A1+4d=17
A1+2d=11
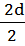 =
= 
d = 3
A1+4[3]=17
A1+12=17-12
A1=5
A13=A1+12d
A13=5+12[3]
A13=5+36
A13 =41
3.
A2=2
A16=-4
A1=?
A2=A1+d
A1+d=2
A16=A1+15d
A1 +15d= -4
Solve the two simultaneously equations by using the elimination method
A1 + d=2
A1 +15d=-4
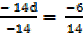
d= 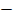

From the 1st equation
A1 +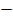
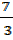 = 2
= 2
A1 = 2+ 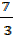
A1 = 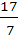
4.
A6= 14
A9=20
A6= A1+5d = 14
A9 = A1+8d=20
Solve the simultaneous equation by using the elimination method
A1+5d= 14
A1+8d=20 ( difference between two equations)
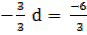
Then d = 2
From 1st equation
A1 +5[2] = 14
A1 +10= 14-10
A1=4
A10= A1+9d
A10=4+9[2]
A 10=4+18
A10=22
An =A1+[n-1]d
An =4[n-1]2
An =4+2n-2
An= 2n+4-2
An=2n+2
5.
A2= 3 x Ab
D= -4
A1=?
An=?
Az = 3 x A6
A 1 +d = 3 x A1+5d
A1+d =3A1+15d
2A1+14d=0
d= -4
2A1+14[-4]=0
2A1+-56= 0
2A1= 56
A1= 28
An = A1+[n-1]d
An= 28+[n-1]-4
An=32-4n
An =32-4n
6.
(a) A3= 0
d= -2
From the formula
A1+2d= 0
A1+2[-2]= 0
A1-4=0
A1= 4
The first term is 4.
b) The general term
An =A1+ [n-1] d
A n =4+[n-1]-2
An = 4+ -2n+2
An = 6-2n
The general term is 6-2n.
7.
A54 =?
100, 97, 94 = A1, A2, A3
A54= A1+53d
d= A2-A1= A3-A2
d= 97-100= 94-97
d= -3
A54= 100+53[-3]
A 54=100 + -159
A54= -59
8.
4, x, y, 20
A 4= 20
A1 +3d=20 , but A1=4
4+3d= 20
3d=16
d = 16/3
A 1, A2, A3, A4
A2 =A1+d
4+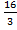
X = 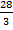
A3 = A1+2d
4+ 2x 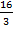
A3 = 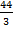
Hence x=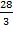 and y=
and y=
9.
A40 =?
A1 =4
A2= 7
A 3= 10
A1+39d=?
d= 7-4= 10-7
d= 3
A1 +39[3]
A1+117
4+117
A40 =121
10.
A1 =4
A2=9
A3=14
d= 9-4= 14-9
d= 5
An =A1+[n-1]d
An =4+[n-1]5
An =4+5n-5
An =5n-1
The nth term is 5n-1.
11.
a) the common difference
A5= 40
A7= 20
A1+ 4d= 40 ………… (1)
A1+ 6d= 20 ………….(2)
Subtracting equation (2) from equation (1) we obtain
-2d=20
d= -10
b) the tenth term
A10= A1+9d,
But A1 +4d=40
A1=80
∴A10=A1+9d
=80-90
A 10=-10
12.
A2= A1+d
A7= A1+6d
A1 +6d = 10
A1+d=7
Solving the simultaneous equations by using the elimination method;
-5d= -3
d = 3/5
A1 +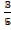 = 7
= 7
A1 =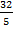
SUM OF THE FIRST n TERMS OF AN ARITHMETIC PROGRESSION
Consider a series with first term A1, common difference d. If the sum n terms is denoted by Sn, then
Sn = A1 + (A1+ d) + (A1 + 2d)+ …+ (A1 – d) + An
+ Sn = An + (An- d) + (An – 2d)+ …+ (A1 + d) + A1
2Sn = (A1+ An) + (A1+ An) + (A1+ An)+ …+ (A1+ An)+ (A1+ An)
There are n terms of (A1+ An) then
2Sn = n(A1+ An)
Sn = n(A1+ An)
2
... The sum of the first n terms of an A.P with first term A1 and the last termAn is given by
Sn =  (A1+ An)
(A1+ An)
But An =A1+ (n-1) d
Thus, from
Sn = 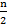 (A1+ An)
(A1+ An)
Sn = 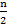 [A1+ A1+ (n-1) d]
[A1+ A1+ (n-1) d]
Sn = 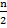 [2A1+ (n-1) d]
[2A1+ (n-1) d]
... therefore, the sum of the first n term of an A.P with the first A1 and the common difference d in given by
Sn = 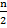 [2A1+ (n-1) d]
[2A1+ (n-1) d]
Where
n =number of terms
A1= first term
An =last term
d= common difference
Example
i) Find sum of the first 5th term where series is 2, 5, 8, 11, 14 first formula
Solution
S5 = [2 + 14]
[2 + 14]
S5 = 40
(ii)Sn =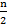 [2A1 + [n-1] d]
[2A1 + [n-1] d]
S5 = [2 x 2 + [5-1] (3)]
[2 x 2 + [5-1] (3)]
S5= 40
Arithmetic Mean
If a, m and b are three consecutive terms of an arithmetic. The common difference
d= M – a
Therefore M- a = b – M
2M = a+ b
M= a + b
2
M is called the arithmetic mean of and b
E.g. Find the arithmetic mean of 3 and 27
M = 3+ 27
2
= 30 = 15
2
GEOMETRIC PROGRESSION (G.P)
Defination:
Geometric progression is a series in which each term after the first is obtained by multiplying the preceding term by the fixed number.
The fixed number is called the common ratio denoted by r.
E.g. 1+2+4+8+16+32+…
3+6+12+24+48
The nth term of Geometric Progression
If n is the number of terms of G.P, the nth term is denoted by Gn and common ratio by r. Then G n+1 = rGn for all natural numbers.
G1 = G1
G2 = G1r
G3 = G2r = G1r.r = G1r2
G4 = G3r = G1r2.r = G1r3
G5 = G4r = G1r3.r = G1r4
G6 = G5r = G1r4.r = G1r5
G7 = G6r = G1r5.r = G1r6
G8 = G7r = G1r6.r = G1r7
The nth term is given by
Gn = G1rn-1
Example1: Write down the eighth term of each of the following.
(a). 2+ 4+ 8+…
(b). 12+ 6+ 3+ …
Solution
(a) The first term G1 =2, the common ratio r=2 and n=8, Then from
Gn = G1rn-1
G8 =(2)(2)8-1
G8 = (2). 27
G8 = 256
(b) The first term G1 =12, the common ratio r=1/2 and n=8, Then from
Gn = G1rn-1
G8 =(12)(1/2)8-1
G8 = (12). (1/2)7
G8 = 12/128 = 3/32
Example2: Find the numbers of terms in the following 1+2+4+8+16+…+512.
Solution
The first term G1 =1, the common ratio r=2 and Gn= 512, Then from
Gn = G1rn-1
512 =(1)(2)n-1
512 = 2n-1
512 = 2n.2-1
512 x 2= 2n
1024= 2n
210 = 2n
n = 10
The sum of the first n terms of a geometrical progression.
Let the sum of first n terms of a G.P be denote by Sn
Sn = G1+ G2+ G3+ G4+…+ Gn
Since the common ratio is r
From
G2 = G1r
G3 = G1r2
G4 = G1r3
…Gn = G1rn-1
Sn = G1+ G1r+ G1r2+ G1r3+…+ Gnrn-1
Multiplying by common ratio r both sides we have
rSn = rG1+ + G1r3+ G1r4+…+ Gnrn
Substract Sn from rSn
rSn – Sn = – G1
Sn(r- 1) = (rn– 1)
Sn =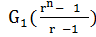 where r ≥ 1 for r≠1
where r ≥ 1 for r≠1
G1= first term of G.P
r= common ratio
Sn = sum of the first nth term
n= number of terms
for r< 1 the formular is given by
sn – rsn = G1 – G1rn
Sn(1-r) = G1(1- rn)
Sn= 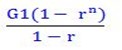 for r<1
for r<1
When r= 1, the sum is simply given by
Sn= G1+G1+G1+G1+G1+G1+…+G1
sn=nG1 for r=1
Example
1. Sum of the first 5th term of G.P where series is 2+4+8+16+32
S5 =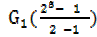
S5 =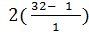
S5 = 62
Sn = 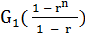
2. The sum of the first n terms of a certain series is given by Sn= 3n-1 show that this series is a G.P
Solution
When
n= 1;
S1 = 31-1 = 3 – 1
= 2
n= 2;
S2 = 32-1 = 9 – 1
= 8
n= 3;
S3 = 33-1 = 27 – 1
= 26
n= 4;
S4 = 34-1 = 81 – 1
= 80
2 + 6 + 18 + 54…
r= 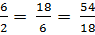
r= 3
Exercise
1. An arithmetic progression has 41 terms. The sum of the first five terms of this A.P is 35 and the sum of the last five terms of the same A.P is 395 find the common difference and the first term.
Solution
S5 = 35
A5 =395
d= ?
A1 =?
S5 = [2A1 + [5-1] d]
[2A1 + [5-1] d]
S5 =  [2A1+4d]
[2A1+4d]
S5= 5A1+10d
35= 5A1+10d … (1)
395=5 A1+190d… (2)
Solve the simultaneous equations
Then the value of d = 2
From the 1st equation
5A1+10d=35
5A1+10[2]=35
5A1+ 20 =35
A1 = 3
Therefore the first term is = 3
2. An arithmetic progression has the first term of 4 and n th term of 256 given that the sum of the nth term is 1280. Find the value of the nth term and common difference
Solution
A1 =4
An =256
Sn =1820
n=?
d=?
Sn =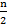 [A1 + An]
[A1 + An]
1820 = 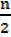 [4 + 256]
[4 + 256]
1820 = n [130]
n= 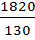
n= 14
Therefore the n term = 14
An = A1+ [n-1]d
An = 4+[14-1]d
256= 4+13d
256-4=13d
252 = 13d
d= 
∴ Common difference =
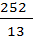
3. The 4th, 5th and6th terms of an A.P are (2x +10), (40x-4) and (8x+40) respectively. Find the first term and the sum of the first 10
A4= 2x+10
A5= 40x-4
A6=8x+40
Solution
A4= A1+3d = (2x + 10)…i
A5 = A1+4d = (40x – 4)…ii
Solve the equations by using elimination method
A1+3d= 2x+10
A1+4d= 40x-4
– d = 2x + 10 – 40x + 4
d= 38x – 14
From 1st equation
A1+3[38x-14] =2x+10
A1+114x – 42=2x +10
A1 = 2x+10 – 114x + 42
A1= -112x +52
Therefore the first terms = -112x+52
S10= 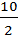 [ 2(-112x+52) +[10-1] 38x-14]
[ 2(-112x+52) +[10-1] 38x-14]
S10=5[-224x+104] +9 [38x-14]
S10=5[-224x+104+342x-126]
S10=5[118x-22]
S10=590x-110
4. The sum of the first n terms of an A.P is given by sn=n[n+3] for all integral values of n. write the first four terms of the series
Solution
When;
n = 1 then sn=n[n+3] =1[1+3]
= 4
n = 2 then =2[2+3]
= 10
n = 3 then =3[3+3]
= 18
n = 4 then =4[4+3]
= 28
The first four terms of the series is 4+6+8+10
5. The sum of the first and fourth terms of an A.P is 18 and the fifth terms is 3 more than the third term. Find the sum of the first 10 terms of this A.P
Solution
A1+A4=18
A5 = ( A1 + 4d )=3( A1 + 2d)
S10 =?
A1+A1+3d =18
2A1+3d=18………..i and ( A1 + 4d )=3( A1 + 2d)
A1 + 4d = 3 A1 + 6d
2 A1 = – 2d
-A1 = d
Substitute the value of d into equation … (i)
2A1+3d=18
2A1+3(-A1)=18
A1 = -18
But -A1 = d this give us d = 18
The sum of the first ten terms Sn =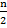 [2A1 + [n-1] d
[2A1 + [n-1] d
S10 =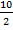 [2 x -18 + [10-1] (18)]
[2 x -18 + [10-1] (18)]
= 630
6. How many terms of the G.P 2+4+8+16…… must be taken to give the sum greater than 10,430?
Solution
G1+G2+G3+G4…………. 2+4+8+16
Sn =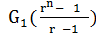
Sn =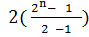
10430 =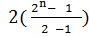
5215 =2n-1
5216 =2n then
n= 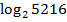
Then more than 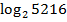 term should be taken to provide the sum greater than 10430
term should be taken to provide the sum greater than 10430
7. In a certain geometrical progression, the third term is 18 and the six term is 486, find the first term and the sum of the first 10 terms of this G.P
Solution
G3= G1 r2 = 18 ………… (1)
G6= G1 r5 = 486……….. (2)
Take equation (2) divide by equation (1)
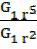 =
= 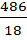
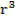 = 27
= 27
r= 3
But 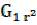 = 18
= 18
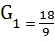
G1 = 2
Sn =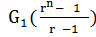 then
then
S10 =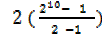
= 2046
The first term is 2
The sum of the first 10 terms is 2046
8. Given that p-2, p-1 and 3p-5 are three consecutive terms of geometric progression find the possible value of p
Solution
R=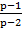 =
= 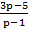
r=[p-1][p-1]=[p-2][3p-5]
r=p2-2p+1=3p2-5p-6p+10
p2-2p+1=3p2-11p+10
p2-2p+1=3p2-11p+10
0=3p 2-p2-11p+2p+10-1
2p2-9p+9=0
From the general quadratic formula
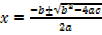
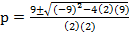
p= 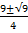
p=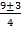
P=3 or p= 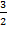
Geometric mean
If a, m and b are consecutive terms of a geometric progression then the common ratio
r= M/a = b/M
M2 = ab
M = 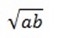
Example: Find the geometric mean of 4 and 16.
from G. M = 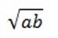
G.M= 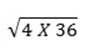
G.M = 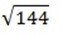
G.M = 12.
5.7. APPLICATION OF A.P AND G.P.
Simple interest is an application of arithmetic progression which is given by;
I = 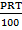
Where
I=simple interest
P=principal
R= rate of interest
T= period of interest
Compound interest is an application of geometric progression it is given by;
An=p+1 or An =p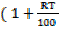 )n
)n
Where
An =an amount at the end of the New Year
R= rate of interest
n= number of years
T=period of interest
p= principal
Example
1. Find the simple interest on Tshs 10,000/= deposited in a bank at the rate of 10% annually for 4 years
Solution
I = 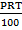
I = 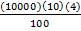
I=1000×4
I=4000
The interest for 4 years will be 4000/=