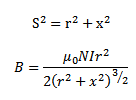FORM 6 PHYSICS: ELECTROMAGNETISM PART 2

 MAGNETIC FIELD AT THE CENTER OF CURRENT CARRYING CIRCULAR COIL

 Consider a circular coil of radius r and carrying current I in the Direction shown in figure

                                                                  

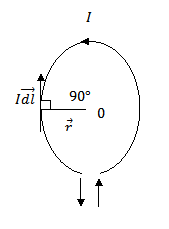
           Suppose  the   loop  lies  in the  plane  of paper it is  desired  to find  the  magnetic field  at  the  centre O of  the  coil

            Suppose  the  entire  circular coil is divided into a  large  number  of  current  elements each of  length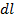

         According  to  Biot – Savant  law, the  magnetic field 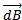 at the centre O of the  coil  due  to current  element  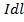is  given  by

  ……………

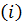
           The  direction  of  dB  is  perpendicular to the  plane  of the  coil and is  Directed  inwards

           Since  each  current  element  contributes  to the magnetic field  in the  same  direction, the  total magnetic field B  at the  centre O can be  found  by integrating equation…………(i)

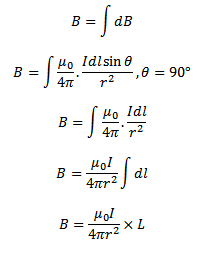
              L- Total length of the coil = 2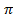r

 

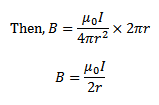
 If the coil has N turns each carrying current in the same direction then contribution of all turn are added up.

 B=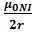

MAGNETIC FIELD DUE TO INFINITELY LONG CONDUCTOR

 

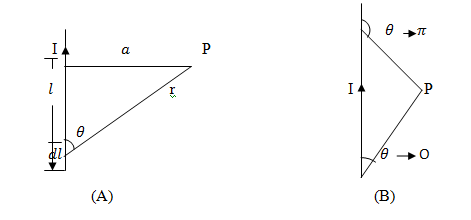
 The flux density dB at P due to the start length dl given by equation as

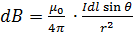
From the figure (A)

          ,
              

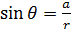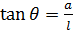
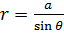
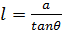
      r =                           

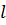         = a cot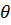

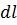        = -a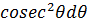 

Substituting for 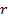 and 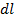 gives

      

       

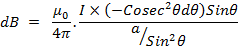
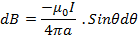
  The total flux density B at P is the sum of the flux densities of all the short lengths and can be found by letting d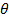→O and integrating over the whole length of the conductor.

       

      

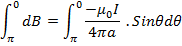
 The  limits  of  the  integration  are 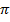 and 0 because  these are  values of 𝜃 at the  ends of the  conductor
    
  

       

       

      

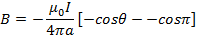
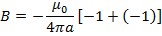
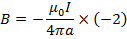
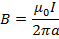
FLUX DENSITY AT ANY POINT ON THE AXIS OF A PLANE CIRCULAR

                         

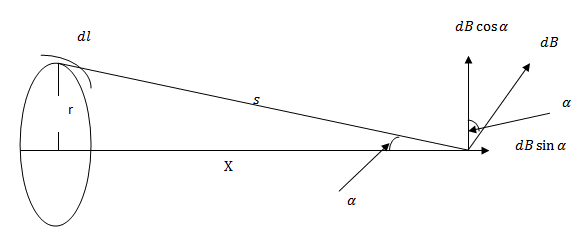
Circular coil with its plane perpendicular to that of the paper

  The  flux  density dB at p due  to the  short length dl of the coil  at  X, where  X is  in  the  plane  of the  paper, is  given by  equation as

   

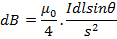
          By symmetry, when all the short lengths 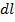 are taken into account the components of magnitude  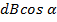sum to zero.

          Each  short length  produces a component of magnitude 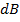Sin α parallel to the  axis and  all those components are  in the  direction shown

          The  total  flux density  is  therefore  in  the  direction of 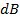Sin α  and  its magnitude B is  given by

             

          

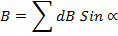
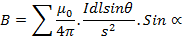
 The radius vector XP of each small length is perpendicular to it, so that 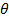=90⁰ and there pore Sin 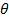= 1

        

Since,

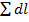= 2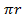(the circumference of the coil)

 

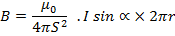
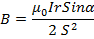 , But  =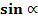

 

 

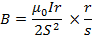
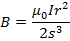
For a coil of N Turns

 

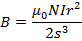
 When S= r

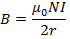
Also from the figure