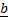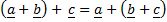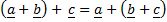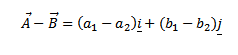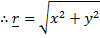LAWS OF VECTORS – ADDITION
A: TRIANGULAR LAW OF VECTORS APPLICATION
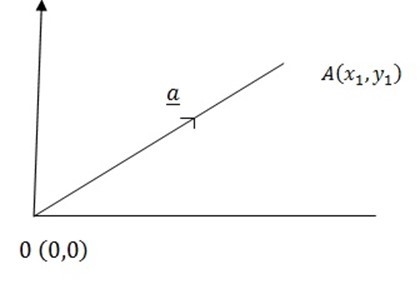
Consider the vector diagram below;
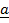 +
+ 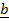 –
– 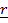 = 0
= 0
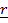 =
= 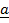 +
+ 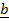
Where r is the resultant vector
B. PARALLELOGRAM LAW OF VECTORS ADDITION
– Consider the vector diagram below
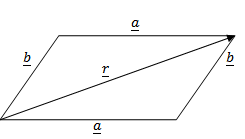
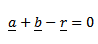
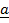 +
+ 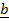 =
= 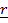 …………………………(i)
…………………………(i)
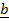 +
+ 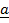 –
– 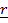 = 0
= 0
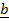 +
+ 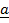 =
=  ……………………………(ii)
……………………………(ii)
From (i) and (ii) above
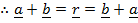
Proved
(ii) Addition of vectors is associative for any three vectors a, b and c
Proof
Consider the vector diagram below;
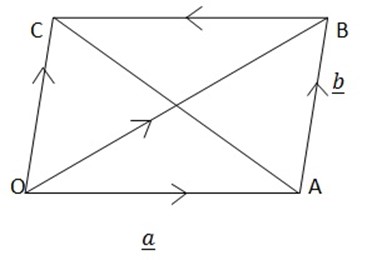
Individual but not considered
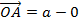
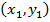 –
–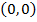
= 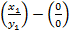
= 
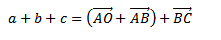
= 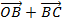
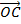 ……(i)
……(i)
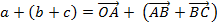
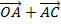
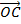 …………………..(ii)
…………………..(ii)
From (i) and (ii) above
= 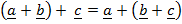
Proved
(iii) For….. of additive identity
For every vector a, we have;
Where;
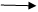 0
0  The null (zero) vector
The null (zero) vector
(iv) Entrance of addictive reverse
For every vector a we have
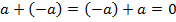
Where
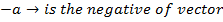
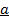 → is the positive of vector
→ is the positive of vector
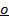 → is the null vector
→ is the null vector
ii. SUBTRACTION OF VECTORS
Suppose two dimensional vectors
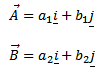
Hence
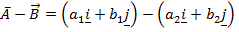
= 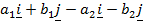
= 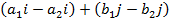
– Suppose three dimensional vectors
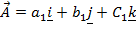
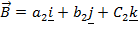
Hence
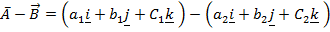
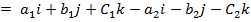
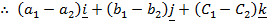
Question 1
1. If 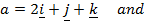
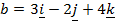
(a Find (i) 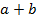
(ii) 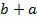
Comment of the results in (a) above
Question 2
Given that
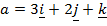
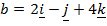
(i) Find 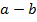
(ii) 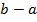
(b) Comment on the results in a above
MAGNITUDE OF A VECTOR
– The magnitude of a vector is a measure of length of the vector.
– – This is denoted by the symbol 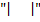
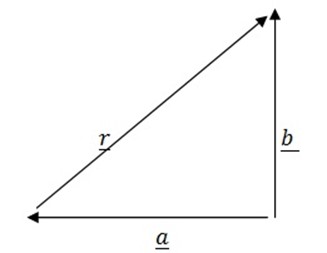
(a) Consider two dimensional vector
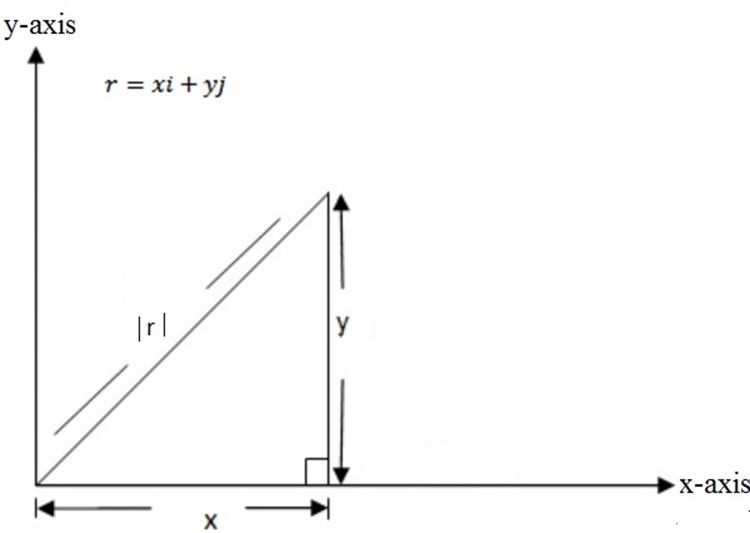
By using Pythagoras theorem
Recall;
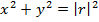
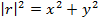
Where
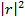 – is the magnitude/ module of the vector r
– is the magnitude/ module of the vector r
(b) Consider three dimensional vector 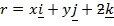
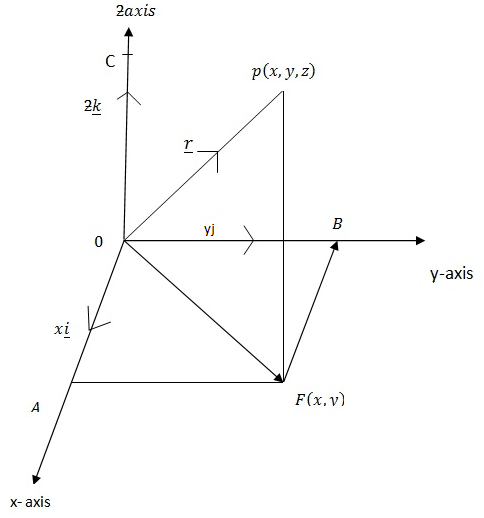
RECTANGULAR RESOLUTION OF A VECTOR
Let:  be three rectangular axes and
be three rectangular axes and 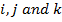 be three unit vectors parallel to
be three unit vectors parallel to 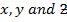 axes respectively.
axes respectively.
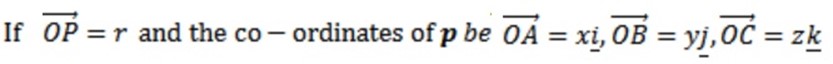
Consider 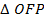
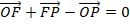
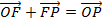
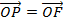 +
+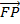
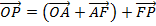
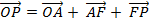
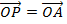 +
+ 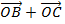
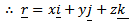
Also consider the right angled OFP
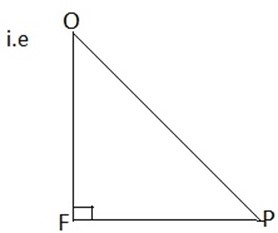
Using Pythagoras theorem
i.e a2 + b2 = c2
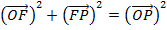

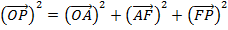
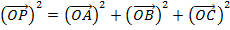
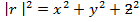
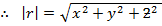
Where
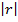 = is the magnitude of the vector
= is the magnitude of the vector 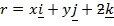
Question
Given that
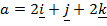
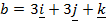
Find 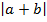

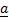 +
+